Misunderstandings
about Understanding in Math Education
Conceptual understanding in math
has served as a dividing line between those who teach in a conventional or
traditional manner (like myself), and those who advocate for progressive
techniques. The progressives/reformers argue that understanding of a procedure
or algorithm must precede the procedure/algorithm itself; failure to do this
results in what some call “math zombies”.
For many concepts in elementary math, understanding builds from
procedures. The student practices the procedure until it is realized
conceptually through familiarity and tactile experience that forges pathways
and connections in the brain. (Furst, 2018). Daniel Ansari (2011), maintains
that procedures and understanding provide mutual support. Rittle-Johnson (2001) supports the push-pull relationship
between understanding and practice of procedure.
Or to put it more plainly, Steve Wilson, a math professor
from Johns Hopkins University at a conference on math education held in Winnipeg
in 2011 stated that “The way mathematicians learn is to learn how to do it
first and then figure out how it works later.”
While
this came as a surprise to some who were on Wilson’s panel, this is a fairly
accurate description of how most of us gain understanding in math: through
familiarity with and practice of procedures.
Nevertheless, the prevailing belief is summarized in statements made by
teachers or school administrators such as “In the past students were taught by
rote; we teach understanding.”
The result of such belief is a
teaching approach in which understanding and process dominate over content. Students are frequently
required to use inefficient methods and to draw pictures, reciting their
understanding at every step. Students
who cannot solve problems in more than one way are believed to lack
understanding. A student unable to
explain in writing how they solved a problem—even in early grades—is taken as
evidence of lack of conceptual understanding. Some students may be held up when
they are clearly ready to move forward and mathematical proficiency is often
sacrificed in the name of understanding.
Levels of Understanding
We are not born as experts—we have to start out as novices. There
are levels of understanding—the level of one’s understanding depends on where
the person is on the spectrum of novice to expert. As students advance along the spectrum from novice to expert, they
acquire more knowledge which is assimilated and connected as the definition
says. The “why” of the procedure is generally
easier to navigate once students are fluent in the particular procedure.
Anyone who has worked with young students you has seen that they gravitate to the “how” or the procedural. Though we may teach the “why”, it is not always grasped at that stage. There is a reason for this “Just tell me” response, given in large part through Cognitive Load Theory (Sweller, et al, 1994, and 2006).
Working memory is where thinking takes place: It is where incoming new information is connected with prior knowledge, and where both are manipulated. It is new information’s “entry ticket” to the long term memory storage. While it plays an important role in thinking, working memory gets overloaded quickly. This is particularly true when trying to juggle many things at once before achieving automaticity of certain procedures leading to information loss. You may have experienced this when someone tells you directions when you’re new to a city. They decide to also tell you some shortcuts and you may say “No, I’m fine, I got it, thanks!”
Learning a procedure or skill is a combination of big picture
understanding and procedural details. Deliberate practice of the procedure
is essential for learning. Repetition brings about automaticity and with that,
a less cluttered working memory. With less clutter, there is more capacity to
make new connections and, ultimately, to understand. Depending on the
procedure, requiring young students to retrieve understanding while mastering
the method can often result in cognitive overload and impede efficiency.
Misunderstandings and Beliefs
The most common misunderstandings about
understanding that I hear include that students should not be taught standard
algorithms before they have the conceptual understanding—it prevents full
understanding of why it works. I also hear that “Getting answers does not
support conceptual understanding.”
Lastly, if a student cannot transfer prior
knowledge to solve never-seen-before problems, that is taken as evidence of a
lack of understanding.
In my experience, a key reason for these misunderstandings is a
tendency to view the world with an adult
lens. As adults, we are experts
who are better problem solvers than our students.
We have a large amount of knowledge. We sometimes forget that what we are teaching is all new to the
students.
Also, one doesn't need to 'deeply
understand' a procedure to do it and do it well. Just as football players and
athletes do numerous drills that look nothing like playing a game of football
or running a marathon, the building blocks of final academic or creative
performance are small, painstaking and deliberate. According to Robert Craigen, a math
professor at University of Manitoba, at the novice level “functional fluency with effective
procedures is the level of understanding that really matters.”
Drilling Understanding—and the Result
Those who believe that understanding must come
before learning a standard algorithm or problem solving procedure frequently
posit that such conceptual understanding helps students. There is some truth to
this belief—namely, it is helpful when the conceptual underpinning is part and parcel to the procedure. For example, in algebra,
understanding the derivation of the rule of adding exponents when multiplying
powers can help students know when to add exponents and when to multiply.
When the concept or derivation is not as closely attached,
however, such as with fractional multiplication and division, insisting on
students showing understanding of the derivation does not provide an obvious
benefit. Nevertheless, a prevailing belief in
education remains that not understanding the concept renders the procedure as a
“rule or trick” with no connection of what is actually going on mathematically.
This belief has led to making students “drill understanding”.
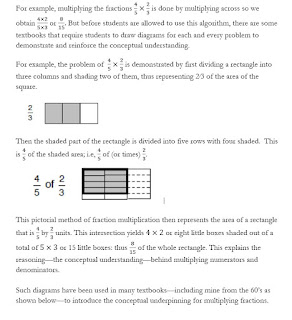
For example, multiplying the fractions  is done by multiplying across so we obtain
is done by multiplying across so we obtain  or
or  But before students
are allowed to use this algorithm, there are some textbooks that require
students to draw diagrams for each and every problem to demonstrate and
reinforce the conceptual understanding.
But before students
are allowed to use this algorithm, there are some textbooks that require
students to draw diagrams for each and every problem to demonstrate and
reinforce the conceptual understanding.
For example, the problem of  is demonstrated by first dividing a rectangle
into three columns and shading two of them, thus representing 2⁄3 of the
area of the square.
is demonstrated by first dividing a rectangle
into three columns and shading two of them, thus representing 2⁄3 of the
area of the square.

Then the shaded part of the rectangle is divided into
five rows with four shaded. This is  of the shaded area;
i.e,
of the shaded area;
i.e,  of (or times)
of (or times) .
. 

This pictorial method of fraction multiplication then
represents the area of a rectangle that is  by
by units. This intersection yields
units. This intersection yields  or eight
little boxes shaded out of a total of
or eight
little boxes shaded out of a total of  or 15
little boxes: thus
or 15
little boxes: thus  of the
whole rectangle. This explains the reasoning—the conceptual
understanding—behind multiplying numerators and denominators.
of the
whole rectangle. This explains the reasoning—the conceptual
understanding—behind multiplying numerators and denominators.
Such diagrams have been used in many textbooks—including mine from
the 60’s as shown below—to introduce the conceptual underpinning for
multiplying fractions.

In my particular book, students used
the area model for, at
most, two fraction multiplication problems. Students were then let loose
to solve more problems using the algorithm. But many textbooks claiming
alignment with the Common Core, require students to draw these type of diagrams
for a full set of problems—in essence drilling understanding.
While the goal of drilling understanding is
to reinforce concepts, it generally leads to what I call “rote
understanding”—exercises that become new procedures to be memorized. Such
drilling forces students to dwell for long periods of time on each problem and
holds up students’ development when they are ready to move forward.
On the other hand, there are
levels of conceptual understanding that are essential—foundational levels. In
the case of fraction multiplication and division, students should know what
each of these operations represent and what kind of problems can be solved with
it. For example: Mrs. Green used  of
of  pounds of sugar to make a
cake. How much sugar did she use?
pounds of sugar to make a
cake. How much sugar did she use?
Given two students, one who knows the derivation of the fraction
multiplication rule, and one who doesn’t, if both see that the solution to the
problem is  and can do the operation
correctly, I cannot tell which student knows the derivation, and which does
not. And at this stage of learning, I am more concerned with their foundational
level of understanding.
and can do the operation
correctly, I cannot tell which student knows the derivation, and which does
not. And at this stage of learning, I am more concerned with their foundational
level of understanding.
Further Questions
In wrapping up this discussion
about misunderstandings about understanding in math, I want to address two
statements that for me raise many questions.
I have heard people say “Calculation is the price we used to
have to pay to do math. It's no longer the case. What we need to learn is the
mathematical understanding.”
And often on the heels of this statement I will hear that
they had done well in math all through elementary school, but when they got to
algebra in high school they hit a wall.
Or, similarly, they did great in high school, and hit a wall with
calculus.
There is much information that we do not have from such
statements.
·
Was
the education they received really devoid of any kind of understanding; that
is, was it all rote?
·
Are
there people who get A’s in math in high school who are really math zombies and
cannot progress to the next level?
·
Are
these complaints limited to those who were educated in the era of traditional
or conventionally taught math?
·
And
of those, how much of what they experienced is due to concepts not explained
well, emphasis on procedures only, and grade inflation?
·
And
to what extent are these problems the result of the obsession over
understanding?
I would be curious to see any research that has been done on
this—either verifying or disproving such notion. In addition, I would also like
to see research conducted in the following areas:
·
For
successful math students in high school and college what did they do that’s
different than those who were successful in math in high school but did not do
well in college math courses
·
What
effect has the emphasis on understanding been on students who have been
identified as having a learning disability?
·
And
a more difficult question: is there evidence that such emphasis has resulted in
students being labeled as having learning disabilities?
·
Finally,
people have told me that those students in lower grades who were “taught
understanding” do better in the long-term than those students for whom the focus
was procedural fluency. Are there studies that support or disprove this?
Based on what I see in the classroom, research that I have
read (see references), and people in the field with whom I have spoken I
believe that attaining procedural fluency and conceptual understanding is an
iterative process of which practice is key. I also strongly believe that whether
understanding or procedure comes first ought to be driven by subject matter and
student need — not by educational ideology.
References:
Ansari, D. (2011). Disorders of the
mathematical brain : Developmental dyscalculia and mathematics anxiety.
Presented at The Art and Science of Math
Education, University of Winnipeg, November 19th 2011. http://mathstats.uwinnipeg.ca/mathedconference/talks/Daniel-Ansari.pdf
Furst, E.
(2018) Understanding ‘Understanding’ in
blog Bridging (Neuro)Science and Education https://sites.google.com/view/efratfurst/understanding-understanding?authuser=0
Geary, D. C., & Menon, V. (in
press). Fact retrieval deficits in mathematical learning disability: Potential
contributions of prefrontal-hippocampal functional organization. In M.
Vasserman, & W. S. MacAllister (Eds.), The Neuropsychology of Learning Disorders: A Handbook for the
Multi-disciplinary Team, New York: Springer
Morgan, P., Farkas, G., MacZuga, S.
(2014). Which instructional practices most help first-grade students with and
without mathematics difficulties?; Educational
Evaluation and Policy Analysis Monthly 201X, Vol. XX, No. X, pp. 1–22.
doi: 10.3102/0162373714536608
National Mathematics Advisory
Panel. (2008). Foundations of success: Final report. U.S. Department of Education. https://www2.ed.gov/about/bdscomm/list/mathpanel/report/final-report.pdf
Rittle-Johnson, B., Siegler, R.S., Alibali,
M.W. (2001). Developing conceptual understanding and procedural skill in
mathematics: An iterative process. Journal
of Educational Psychology, Vol. 93, No. 2, 346-362. doi:
10.1037//0022-0063.93.2.346
Sweller, P. (1994) Cognitive load
theory, learning difficulty, and instructional design. Leaming and Instruction, Vol. 4, pp. 293-312
Sweller, P. (2006). The worked
example effect and human cognition. Learning
and Instruction, 16(2) 165–169

8 comments:
In college, majoring in aerospace engineering, I did seven semesters of advanced math - Calc 1-3, Differential Equations 1 (ODEs) and DiffEqs 2 (PDEs), Advanced PDEs, and Tensor Calc + Intro to Calculus of Variations. Plus a numerical methods class, focusing on linear algebra. I got A’s in all of them. And in every one, the only way I achieved understanding was to do enough problems to develop understanding about what was really going on behind the scenes. “Oh, so that’s what integration by parts is really doing!” “Ah, now I get what the complex exponential solution to the 2nd order linear ODE means!”
We really do know what works, and we know why - it’s all because of how we hijack our brains to perform stuff we never evolved for. How much longer are we going to stand for nonsense in math instruction?
My kid hit a wall when he got to calc this year, but it is because math came so easily to him in the previous years, that he never had to work at it. He could blow off the homework (which was usually just checked done and not corrected) and still do well on tests.
This year, blowing off the work was nearly disastrous. After tanking a couple tests, we sat him down over spring break and made him do every problem he had been assigned. He got a 94% on the test the following week. He kept saying he didn't understand what was going on, but by the end, while working the problems, he figured it out.
Who decides what concepts are foundational?
That is the key question. One third grade teacher does the 9s finger trick and calls it good for multiplication. The other teaches all the properties in the process of learning the table. One sixth grade teacher teaches concepts with practice, the other does invert and multiply. One algebra teacher uses properties, one teaches FOIL. Which one do you want your thinking child in? In the age of disparate impact, what are you going to accept - the choice that puts your child in remedial community college, or the one that leads to beginning an Engineering program done with Diff Eq?
Greetings from the Antipodes,
Is there a link to the original document (as a word or pdf) available? I would love to read the original if possible.
Thanks.
I don't have a link, he emailed me the Word doc.
"Which one do you want your thinking child in? In the age of disparate impact, what are you going to accept - the choice that puts your child in remedial community college, or the one that leads to beginning an Engineering program done with Diff Eq?"
Many teachers teach both the conceptual understanding and the procedures. To insist on understanding (i.e, "concepts with practice") is like the example I gave in the article--requiring the student to reproduce an inefficient diagram or process in the name of gaining understanding. At the novice level functional fluency with effective procedures is the level of understanding that really matters. It does not obviate growth and eventual understanding or predetermine that the student will not get into an engineering program.
The article was an abridged version of a talk I gave. You can download the slides, which when viewed in "Notes" format contain the script that is associated with each slide.
Can be downloaded from this link: https://researched.org.uk/?ddownload=21069
"Who decides what concepts are foundational?"
Mathemeticians, not ED school pedagogues who care more about full inclusion and improving low average statistics.
"One third grade teacher does the 9s finger trick and calls it good for multiplication. The other teaches all the properties in the process of learning the table. One sixth grade teacher teaches concepts with practice, the other does invert and multiply. One algebra teacher uses properties, one teaches FOIL. Which one do you want your thinking child in?
One that is based on a proper math textbook and STEM-level expectations, not CCSS "proficiency" or the whim of teachers who have absolutly no certification in math - one that enforces individual weekly mastery, not silly in-class group learning where the kids with mastery enforcement at home excel and the others go along for the fun ride that makes teachers think that real understanding is being achieved by all. I would be their biggest fan if there was any shred of evidence that it did anything more than float a few more boats to a NON-STEM level of CCSS proficiency.
I got to calculus in my "traditional" math youth with absolutely no help from my parents. My son and all of his STEM-prepared friends had to have help from parents or tutors. This was not a problem for their traditional AP-track math in high school. The problem is in the fuzzy world of K-6 math that tries to hide NON-STEM level K-6 math behind fake claims of "understanding." This is a fundamental systemic failure and kids and parents have to pick up the pieces. You can't change to wider full-inclusion population and then claim to do better for all with lower expectations. This ED school "understanding" talk is all just misdirection.
"In the age of disparate impact, what are you going to accept - the choice that puts your child in remedial community college, or the one that leads to beginning an Engineering program done with Diff Eq?"
The current low expectation K-12 CCSS math, by definition, leads to NON-STEM no remediation at the CC level. There are no other options offered by CCSS. Proper math that focuses on mastery of individual skill problem sets weekly leads to proper AP/IB classes in high school, and that leads to an engineering degree in math. That K-6 help and expectations have now been handed over to parents and tutors. Separate out from the algebra in 8th grade track those who had mastery help from parents or tutors. How many do you have left? That's an easy research project to do.
CCSS math defines a one-slope path from Kindergarten to no remediation for a college algebra course at the community college level. CCSS is NON-STEM by definition. There is no path to prepare for the traditional high school AP/IB math tracks that are at a much higher slope. The College Board knows that this is a problem and has instituted their Pre-AP algebra course for ninth grade that focuses on mastery of skills. They talk about social justice, but where is the social justice in their support of low expectations for K-8 and their very high expectation that ninth grade algebra students will magically do well in AP calculus as seniors - taking 5 tough traditional math classes in 4 years? This all just hides the K-8 differentiated instruction at home by parents who will not let their kids be unprepared.
Post a Comment